Express The Given Hindu Arabic Numeral In Expanded Form
Express The Given Hindu Arabic Numeral In Expanded Form - ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution 100% (1 rating) transcribed image text: This problem has been solved!. Do not perform the calculation.). Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. Web the evolution of a system. (5 × 103) + (3 × 102) + (2 × 101) + (5 × 100) = 5,325 word form: 5,000 + 300 + 20 + 5 = 5,325 expanded factors form: 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. (5x103) + (0x102) + (0x104) + (8x1) (5x103) + (0x102) +. This problem has been solved!. 5,000 + 300 + 20 + 5 = 5,325 expanded factors form: The modern system of counting and computing isn’t. 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution (5 × 1,000) + (3 × 100) + (2 × 10) + (5 ×. The modern system of counting and computing isn’t. You'll get a detailed solution from a subject matter expert that. (3 × 1 0 2) + (8 × 1 0 1) + (5 × 1) \left(3 \times 10^{2}\right)+\left(8 \times 10^{1}\right)+(5 \times 1) (3 × 1 0 2) + (8 × 1 0. (5 × 1,000) + (3 × 100) + (2. 5,000 + 300 + 20 + 5 = 5,325 expanded factors form: V this problem has been solved! 7647 7647 = (use the multiplication symbol in the math palette as needed. Do not perform the calculation.). 100% (1 rating) transcribed image text: This problem has been solved!. Web the evolution of a system. (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. You'll get a detailed solution from a subject matter expert that. (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. We start by showing all powers of 10, starting with the highest exponent. Web the evolution of a system. Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. (3 × 1 0 2) + (8 × 1 0 1) + (5 × 1) \left(3 \times 10^{2}\right)+\left(8 \times 10^{1}\right)+(5 \times 1) (3 × 1 0 2) + (8 × 1 0. 5,000 + 300 + 20. This problem has been solved!. We start by showing all powers of 10, starting with the highest exponent given. (1× 102)+ (5× 101)+ (7× 1) 13 35 130 157. (3 × 1 0 2) + (8 × 1 0 1) + (5 × 1) \left(3 \times 10^{2}\right)+\left(8 \times 10^{1}\right)+(5 \times 1) (3 × 1 0 2) + (8 × 1. Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. 100% (1 rating) transcribed image text: You'll get a detailed solution from a subject matter expert that. We start by showing all powers of 10, starting with the highest exponent given. Web the evolution of a system. (5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 expanded exponential form: (1× 102)+ (5× 101)+ (7× 1) 13 35 130 157. 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. This problem has been solved!. ( 9 × 1 0 1 ) + ( 4 ×. 100% (1 rating) transcribed image text: Web the evolution of a system. This problem has been solved!. ( 9 × 1 0 1 ) + ( 4 × 1 ) \left(9 \times 10^{1}\right)+(4 \times 1) ( 9 × 1 0 1 ) + ( 4 × 1 ) solution ( 7 × 1 0 1 ) + ( 3 × 1 ) \left(7 \times 10^{1}\right)+(3 \times 1) ( 7 × 1 0 1 ) + ( 3 × 1 ) solution (1× 102)+ (5× 101)+ (7× 1) 13 35 130 157. (5x103) + (0x102) + (0x104) + (8x1) (5x103) + (0x102) +. 32,714 32,714 = 1 (use the multiplication symbol in the math palette as needed. 5,000 + 300 + 20 + 5 = 5,325 expanded factors form: (3 × 1 0 2) + (8 × 1 0 1) + (5 × 1) \left(3 \times 10^{2}\right)+\left(8 \times 10^{1}\right)+(5 \times 1) (3 × 1 0 2) + (8 × 1 0. Do not perform the calculation.). 7647 7647 = (use the multiplication symbol in the math palette as needed. You'll get a detailed solution from a subject matter expert that. Web you'll get a detailed solution from a subject matter expert that helps you learn core concepts. Parenthesis four times ten to the fourth power close parenthesis, plus parenthesis seven. (7 × 103) + (5 × 101) + (4 × 1).
Answered Express each expanded form as a… bartleby

PPT 4.1 PowerPoint Presentation, free download ID5936567

Writing HinduArabic Numerals in Expanded Form
[Solved] Express the given expanded numeral as a HinduArabic numeral
![[ANSWERED] Use the table to write the given Hindu Arabic numera](https://i2.wp.com/media.kunduz.com/media/sug-question-candidate/20230127203142998938-3653173_gtppalU.jpg?h=512)
[ANSWERED] Use the table to write the given Hindu Arabic numera

The Hindu—Arabic Number System and Roman Numerals (2023)
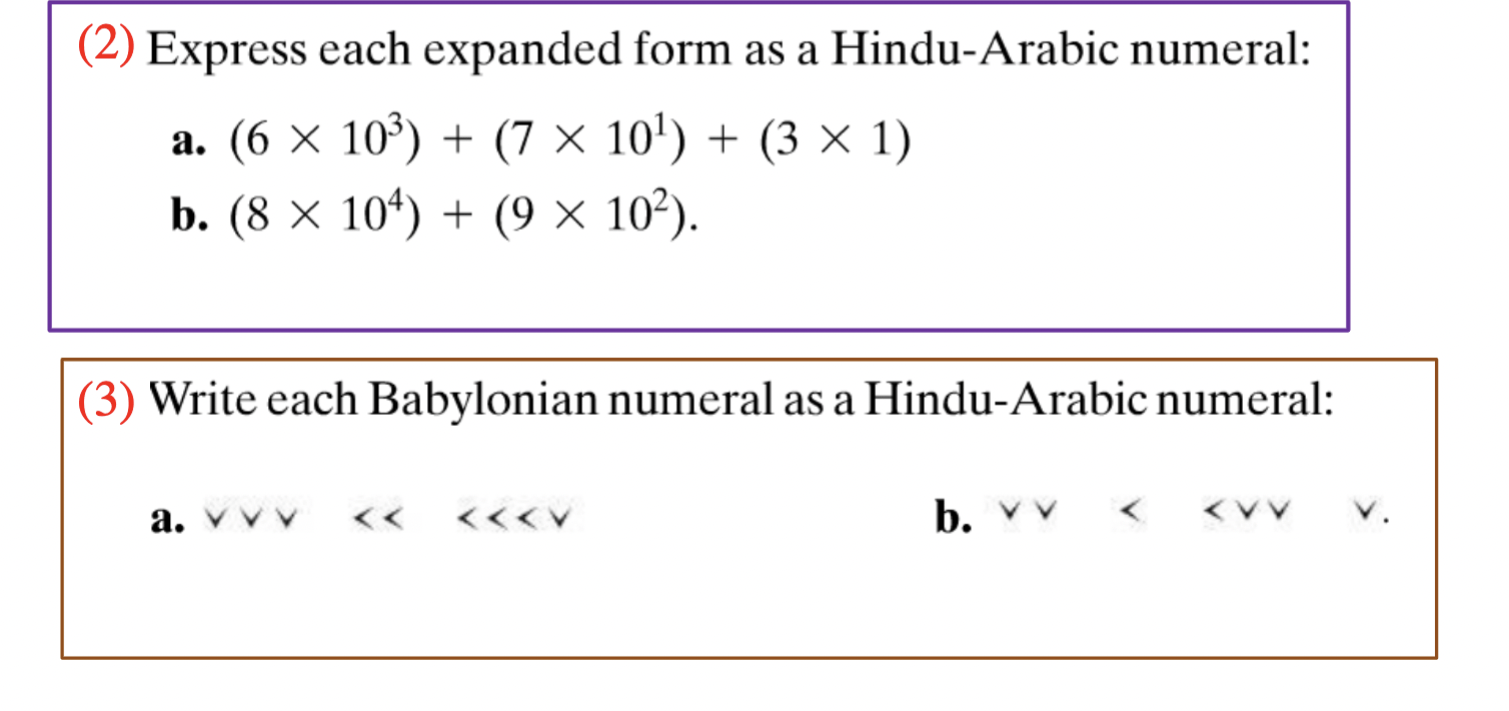
Solved (2) Express each expanded form as a HinduArabic

Writing HinduArabic Numerals in Expanded Form
[Solved] Express the given HinduArabic numeral in expanded form. 907 O

Writing HinduArabic Numerals in Expanded Form
The Modern System Of Counting And Computing Isn’t.
(5 × 103) + (3 × 102) + (2 × 101) + (5 × 100) = 5,325 Word Form:
V This Problem Has Been Solved!
(5 × 1,000) + (3 × 100) + (2 × 10) + (5 × 1) = 5,325 Expanded Exponential Form:
Related Post: